This article includes a list of general references, but it lacks sufficient corresponding inline citations. (May 2020) |
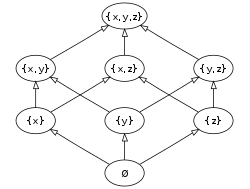
In mathematics, the axiom of power set[1] is one of the Zermelo–Fraenkel axioms of axiomatic set theory. It guarantees for every set the existence of a set , the power set of , consisting precisely of the subsets of . By the axiom of extensionality, the set is unique.
The axiom of power set appears in most axiomatizations of set theory. It is generally considered uncontroversial, although constructive set theory prefers a weaker version to resolve concerns about predicativity.
- ^ "Axiom of power set | set theory | Britannica". www.britannica.com. Retrieved 2023-08-06.

