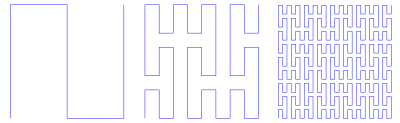
In mathematical analysis, a space-filling curve is a curve whose range reaches every point in a higher dimensional region, typically the unit square (or more generally an n-dimensional unit hypercube). Because Giuseppe Peano (1858–1932) was the first to discover one, space-filling curves in the 2-dimensional plane are sometimes called Peano curves, but that phrase also refers to the Peano curve, the specific example of a space-filling curve found by Peano.
The closely related FASS curves (approximately space-Filling, self-Avoiding, Simple, and Self-similar curves) can be thought of as finite approximations of a certain type of space-filling curves.[1][2][3][4][5][6]
- ^ Przemyslaw Prusinkiewicz and Aristid Lindenmayer. "The Algorithmic Beauty of Plants". 2012. p. 12
- ^ Jeffrey Ventrella. "Brainfilling Curves - A Fractal Bestiary". 2011. p. 43
- ^ Marcia Ascher. "Mathematics Elsewhere: An Exploration of Ideas Across Cultures". 2018. p. 179.
- ^ "Fractals in the Fundamental and Applied Sciences". 1991. p. 341-343.
- ^ Przemyslaw Prusinkiewicz; Aristid Lindenmayer; F. David Fracchia. "Synthesis of Space-Filling Curves on the Square Grid". 1989.
- ^ "FASS-curve". D. Frettlöh, E. Harriss, F. Gähler: Tilings encyclopedia, https://tilings.math.uni-bielefeld.de/