
In differential geometry a translation surface is a surface that is generated by translations:
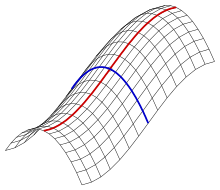
- For two space curves with a common point , the curve is shifted such that point is moving on . By this procedure curve generates a surface: the translation surface.
If both curves are contained in a common plane, the translation surface is planar (part of a plane). This case is generally ignored.


Simple examples:
- Right circular cylinder: is a circle (or another cross section) and is a line.
- The elliptic paraboloid can be generated by and (both curves are parabolas).
- The hyperbolic paraboloid can be generated by (parabola) and (downwards open parabola).
Translation surfaces are popular in descriptive geometry[1][2] and architecture,[3] because they can be modelled easily.
In differential geometry minimal surfaces are represented by translation surfaces or as midchord surfaces (s. below).[4]
The translation surfaces as defined here should not be confused with the translation surfaces in complex geometry.
- ^ H. Brauner: Lehrbuch der Konstruktiven Geometrie, Springer-Verlag, 2013,ISBN 3709187788, 9783709187784, p. 236
- ^ Fritz Hohenberg: Konstruktive Geometrie in der Technik, Springer-Verlag, 2013, ISBN 3709181488, 9783709181485, p. 208
- ^ Hans Schober: Transparente Schalen: Form, Topologie, Tragwerk, John Wiley & Sons, 2015, ISBN 343360598X, 9783433605981, S. 74
- ^ Wilhelm Blaschke, Kurt Reidemeister: Vorlesungen über Differentialgeometrie und geometrische Grundlagen von Einsteins Relativitätstheorie II: Affine Differentialgeometrie, Springer-Verlag, 2013,ISBN 364247392X, 9783642473920, p. 94









