| Truncated order-7 triangular tiling | |
|---|---|
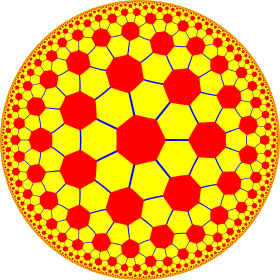 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic uniform tiling |
| Vertex configuration | 7.6.6 |
| Schläfli symbol | t{3,7} |
| Wythoff symbol | 2 7 | 3 |
| Coxeter diagram | |
| Symmetry group | [7,3], (*732) |
| Dual | Heptakis heptagonal tiling |
| Properties | Vertex-transitive |
In geometry, the order-7 truncated triangular tiling, sometimes called the hyperbolic soccerball,[1] is a semiregular tiling of the hyperbolic plane. There are two hexagons and one heptagon on each vertex, forming a pattern similar to a conventional soccer ball (truncated icosahedron) with heptagons in place of pentagons. It has Schläfli symbol of t{3,7}.