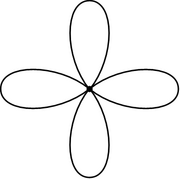
In mathematics, a bouquet graph , for an integer parameter , is an undirected graph with one vertex and edges, all of which are self-loops. It is the graph-theoretic analogue of the topological rose, a space of circles joined at a point. When the context of graph theory is clear, it can be called more simply a bouquet.[1]
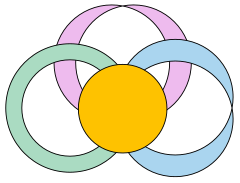
Although bouquets have a very simple structure as graphs, they are of some importance in topological graph theory because their graph embeddings can still be non-trivial. In particular, every cellularly embedded graph can be reduced to an embedded bouquet by a partial duality applied to the edges of any spanning tree of the graph,[2] or alternatively by contracting the edges of any spanning tree.
In graph-theoretic approaches to group theory, every Cayley–Serre graph (a variant of Cayley graphs with doubled edges) can be represented as the covering graph of a bouquet.[3]



