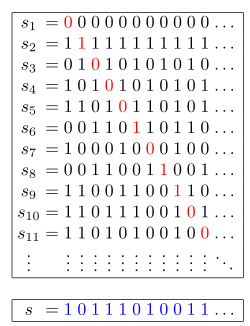
Cantor's diagonal argument (among various similar names[note 1]) is a mathematical proof that there are infinite sets which cannot be put into one-to-one correspondence with the infinite set of natural numbers – informally, that there are sets which in some sense contain more elements than there are positive integers. Such sets are now called uncountable sets, and the size of infinite sets is treated by the theory of cardinal numbers, which Cantor began.
Georg Cantor published this proof in 1891,[1][2]: 20– [3] but it was not his first proof of the uncountability of the real numbers, which appeared in 1874.[4][5] However, it demonstrates a general technique that has since been used in a wide range of proofs,[6] including the first of Gödel's incompleteness theorems[2] and Turing's answer to the Entscheidungsproblem. Diagonalization arguments are often also the source of contradictions like Russell's paradox[7][8] and Richard's paradox.[2]: 27
Cite error: There are <ref group=note> tags on this page, but the references will not show without a {{reflist|group=note}} template (see the help page).
- ^ Georg Cantor (1891). "Ueber eine elementare Frage der Mannigfaltigkeitslehre". Jahresbericht der Deutschen Mathematiker-Vereinigung. 1: 75–78. English translation: Ewald, William B., ed. (1996). From Immanuel Kant to David Hilbert: A Source Book in the Foundations of Mathematics, Volume 2. Oxford University Press. pp. 920–922. ISBN 0-19-850536-1.
- ^ a b c Keith Simmons (30 July 1993). Universality and the Liar: An Essay on Truth and the Diagonal Argument. Cambridge University Press. ISBN 978-0-521-43069-2.
- ^ Rudin, Walter (1976). Principles of Mathematical Analysis (3rd ed.). New York: McGraw-Hill. p. 30. ISBN 0070856133.
- ^ Gray, Robert (1994), "Georg Cantor and Transcendental Numbers" (PDF), American Mathematical Monthly, 101 (9): 819–832, doi:10.2307/2975129, JSTOR 2975129
- ^ Bloch, Ethan D. (2011). The Real Numbers and Real Analysis. New York: Springer. p. 429. ISBN 978-0-387-72176-7.
- ^ Sheppard, Barnaby (2014). The Logic of Infinity (illustrated ed.). Cambridge University Press. p. 73. ISBN 978-1-107-05831-6. Extract of page 73
- ^ Russell's paradox. Stanford encyclopedia of philosophy. 2021.
- ^ Bertrand Russell (1931). Principles of mathematics. Norton. pp. 363–366.