Conformally flat manifold

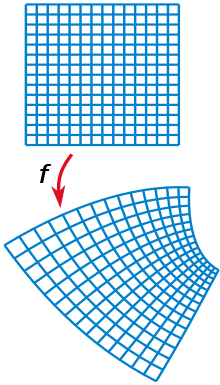
A (pseudo-)Riemannian manifold is conformally flat if each point has a neighborhood that can be mapped to flat space by a conformal transformation.

In practice, the metric ${\displaystyle g}$ of the manifold ${\displaystyle M}$ has to be conformal to the flat metric ${\displaystyle \eta }$, i.e., the geodesics maintain in all points of ${\displaystyle M}$ the angles by moving from one to the other, as well as keeping the null geodesics unchanged,^[1] that means there exists a function ${\displaystyle \lambda (x)}$ such that ${\displaystyle g(x)=\lambda ^{2}(x)\,\eta }$, where ${\displaystyle \lambda (x)}$ is known as the conformal factor and ${\displaystyle x}$ is a point on the manifold.

More formally, let ${\displaystyle (M,g)}$ be a pseudo-Riemannian manifold. Then ${\displaystyle (M,g)}$ is conformally flat if for each point ${\displaystyle x}$ in ${\displaystyle M}$, there exists a neighborhood ${\displaystyle U}$ of ${\displaystyle x}$ and a smooth function ${\displaystyle f}$ defined on ${\displaystyle U}$ such that ${\displaystyle (U,e^{2f}g)}$ is flat (i.e. the curvature of ${\displaystyle e^{2f}g}$ vanishes on ${\displaystyle U}$). The function ${\displaystyle f}$ need not be defined on all of ${\displaystyle M}$.

Some authors use the definition of locally conformally flat when referred to just some point ${\displaystyle x}$ on ${\displaystyle M}$ and reserve the definition of conformally flat for the case in which the relation is valid for all ${\displaystyle x}$ on ${\displaystyle M}$.