Contour line

This article is about lines of equal value in maps and diagrams. For more meanings of the word "contour", see Contour (disambiguation).
The bottom part of the diagram shows some contour lines with a straight line running through the location of the maximum value. The curve at the top represents the values along that straight line.
A three-dimensional surface, whose contour graph is below.
A two-dimensional contour graph of the three-dimensional surface in the above picture.

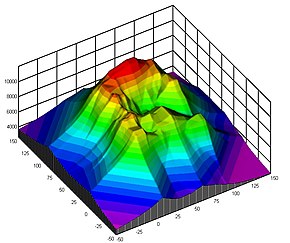
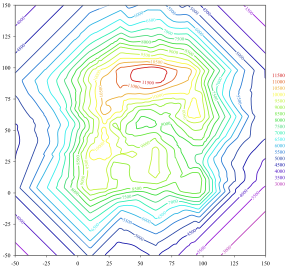
A contour line (also isoline, isopleth, isoquant or isarithm) of a function of two variables is a curve along which the function has a constant value, so that the curve joins points of equal value.[1][2] It is a plane section of the three-dimensional graph of the function parallel to the -plane. More generally, a contour line for a function of two variables is a curve connecting points where the function has the same particular value.[2]

In cartography, a contour line (often just called a "contour") joins points of equal elevation (height) above a given level, such as mean sea level.[3] A contour map is a map illustrated with contour lines, for example a topographic map, which thus shows valleys and hills, and the steepness or gentleness of slopes.[4] The contour interval of a contour map is the difference in elevation between successive contour lines.[5]

The gradient of the function is always perpendicular to the contour lines. When the lines are close together the magnitude of the gradient is large: the variation is steep. A level set is a generalization of a contour line for functions of any number of variables.

Contour lines are curved, straight or a mixture of both lines on a map describing the intersection of a real or hypothetical surface with one or more horizontal planes. The configuration of these contours allows map readers to infer the relative gradient of a parameter and estimate that parameter at specific places. Contour lines may be either traced on a visible three-dimensional model of the surface, as when a photogrammetrist viewing a stereo-model plots elevation contours, or interpolated from the estimated surface elevations, as when a computer program threads contours through a network of observation points of area centroids. In the latter case, the method of interpolation affects the reliability of individual isolines and their portrayal of slope, pits and peaks.[6]

  1. ^ Courant, Richard, Herbert Robbins, and Ian Stewart. What Is Mathematics?: An Elementary Approach to Ideas and Methods. New York: Oxford University Press, 1996. p. 344.
  2. ^ a b Hughes-Hallett, Deborah; McCallum, William G.; Gleason, Andrew M. (2013). Calculus : Single and Multivariable (6 ed.). John wiley. ISBN 978-0470-88861-2.
  3. ^ "Definition of contour line". Dictionary.com. Retrieved 2022-04-04.
  4. ^ "Definition of CONTOUR MAP". Merriam-Webster. Retrieved 2022-04-04.
  5. ^ Tracy, John C. Plane Surveying; A Text-Book and Pocket Manual. New York: J. Wiley & Sons, 1907. p. 337.
  6. ^ Davis, John C., 1986, Statistics and data analysis in geology, Wiley ISBN 0-471-08079-9