Diesis

This article is about music. For other uses, see Diesis (disambiguation).
Diesis on C Playⓘ.
Diesis as three just major thirds.
Diesis (128:125) demonstration
The octave C-C′, the three justly tuned major thirds C-E-G♯-B♯ and the descending diesis C′-B♯ are played (see example).
Problems playing this file? See media help.

In classical music from Western culture, a diesis (/ˈdaɪəsɪs/ DY-ə-siss or enharmonic diesis, plural dieses (/ˈdaɪəsiz/ DY-ə-seez),[1] or "difference"; Greek: δίεσις "leak" or "escape"[2][a] is either an accidental (see sharp), or a very small musical interval, usually defined as the difference between an octave (in the ratio 2:1) and three justly tuned major thirds (tuned in the ratio 5:4), equal to 128:125 or about 41.06 cents. In 12-tone equal temperament (on a piano for example) three major thirds in a row equal an octave, but three justly-tuned major thirds fall quite a bit narrow of an octave, and the diesis describes the amount by which they are short. For instance, an octave (2:1) spans from C to C′, and three justly tuned major thirds (5:4) span from C to B♯ (namely, from C, to E, to G♯, to B♯). The difference between C-C′ (2:1) and C-B♯ (125:64) is the diesis (128:125). Notice that this coincides with the interval between B♯ and C′, also called a diminished second.

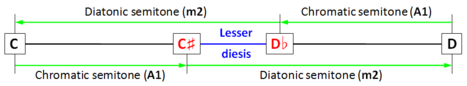
As a comma, the above-mentioned 128:125 ratio is also known as the lesser diesis, enharmonic comma, or augmented comma.

Many acoustics texts use the term greater diesis[2] or diminished comma for the difference between an octave and four justly tuned minor thirds (tuned in the ratio 6:5), which is equal to three syntonic commas minus a schisma, equal to 648:625 or about 62.57 cents (almost one 63.16 cent step-size in 19 equal temperament). Being larger, this diesis was termed the "greater" while the 128:125 diesis (41.06 cents) was termed the "lesser".[3][failed verification]

Diesis defined in quarter-comma meantone as a diminished second (m2 − A1 ≈ 117.1 − 76.0 ≈ 41.1 cents), or an interval between two enharmonically equivalent notes (from D♭ to C♯). Playⓘ
  1. ^ "diesis". American Heritage Dictionary – via ahdictionary.com.
  2. ^ a b c Benson, Dave (2006). Music: A mathematical offering. p. 171. ISBN 0-521-85387-7.
  3. ^ A. B. (2003). "Diesis". In Randel, D. M. (ed.). The Harvard Dictionary of Music (4th ed.). Cambridge, MA: Belknap Press. p. 241.


Cite error: There are <ref group=lower-alpha> tags or {{efn}} templates on this page, but the references will not show without a {{reflist|group=lower-alpha}} template or {{notelist}} template (see the help page).