Monotone likelihood ratio

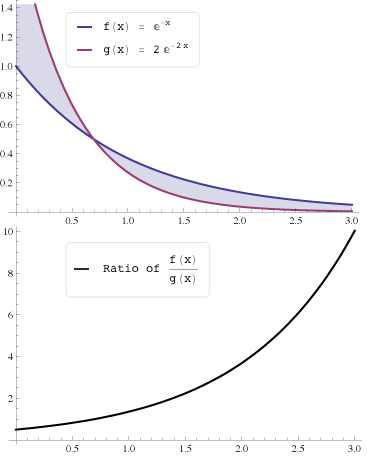
A monotonic likelihood ratio in distributions ${\displaystyle \ f(x)\ }$ and ${\displaystyle \ g(x)\ }$

The ratio of the density functions above is monotone in the parameter ${\displaystyle \ x\ ,}$ so ${\displaystyle \ {\frac {\ f(x)\ }{g(x)}}\ }$ satisfies the monotone likelihood ratio property.

In statistics, the monotone likelihood ratio property is a property of the ratio of two probability density functions (PDFs). Formally, distributions ${\displaystyle \ f(x)\ }$ and ${\displaystyle \ g(x)\ }$ bear the property if

${\displaystyle \ {\text{for every }}x_{2}>x_{1},\quad {\frac {f(x_{2})}{\ g(x_{2})\ }}\geq {\frac {f(x_{1})}{\ g(x_{1})\ }}\ }$

that is, if the ratio is nondecreasing in the argument ${\displaystyle x}$.

If the functions are first-differentiable, the property may sometimes be stated

${\displaystyle {\frac {\ \partial }{\ \partial x}}\left({\frac {f(x)}{\ g(x)\ }}\right)\geq 0\ }$

For two distributions that satisfy the definition with respect to some argument ${\displaystyle \ x\ ,}$ we say they "have the MLRP in ${\displaystyle \ x~.}$" For a family of distributions that all satisfy the definition with respect to some statistic ${\displaystyle \ T(X)\ ,}$ we say they "have the MLR in ${\displaystyle \ T(X)~.}$"