Osculating circle

"Kissing circles" redirects here. For Descartes' theorem on mutually tangent (kissing) circles, see Descartes' theorem.
An osculating circle
Osculating circles of the Archimedean spiral, nested by the Tait–Kneser theorem. "The spiral itself is not drawn: we see it as the locus of points where the circles are especially close to each other."[1]

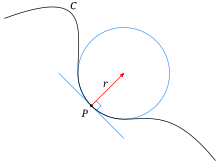
An osculating circle is a circle that best approximates the curvature of a curve at a specific point. It is tangent to the curve at that point and has the same curvature as the curve at that point.[2] The osculating circle provides a way to understand the local behavior of a curve and is commonly used in differential geometry and calculus.

More formally, in differential geometry of curves, the osculating circle of a sufficiently smooth plane curve at a given point p on the curve has been traditionally defined as the circle passing through p and a pair of additional points on the curve infinitesimally close to p. Its center lies on the inner normal line, and its curvature defines the curvature of the given curve at that point. This circle, which is the one among all tangent circles at the given point that approaches the curve most tightly, was named circulus osculans (Latin for "kissing circle") by Leibniz.

The center and radius of the osculating circle at a given point are called center of curvature and radius of curvature of the curve at that point. A geometric construction was described by Isaac Newton in his Principia:

There being given, in any places, the velocity with which a body describes a given figure, by means of forces directed to some common centre: to find that centre.

— Isaac Newton, Principia; PROPOSITION V. PROBLEM I.
  1. ^ Cite error: The named reference gtt was invoked but never defined (see the help page).
  2. ^ "12.4 Arc Length and Curvature". Retrieved 2023-09-19.