Sums of three cubes

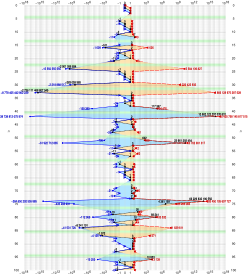
In the mathematics of sums of powers, it is an open problem to characterize the numbers that can be expressed as a sum of three cubes of integers, allowing both positive and negative cubes in the sum. A necessary condition for an integer ${\displaystyle n}$ to equal such a sum is that ${\displaystyle n}$ cannot equal 4 or 5 modulo 9, because the cubes modulo 9 are 0, 1, and −1, and no three of these numbers can sum to 4 or 5 modulo 9.^[1] It is unknown whether this necessary condition is sufficient.

Variations of the problem include sums of non-negative cubes and sums of rational cubes. All integers have a representation as a sum of rational cubes, but it is unknown whether the sums of non-negative cubes form a set with non-zero natural density.